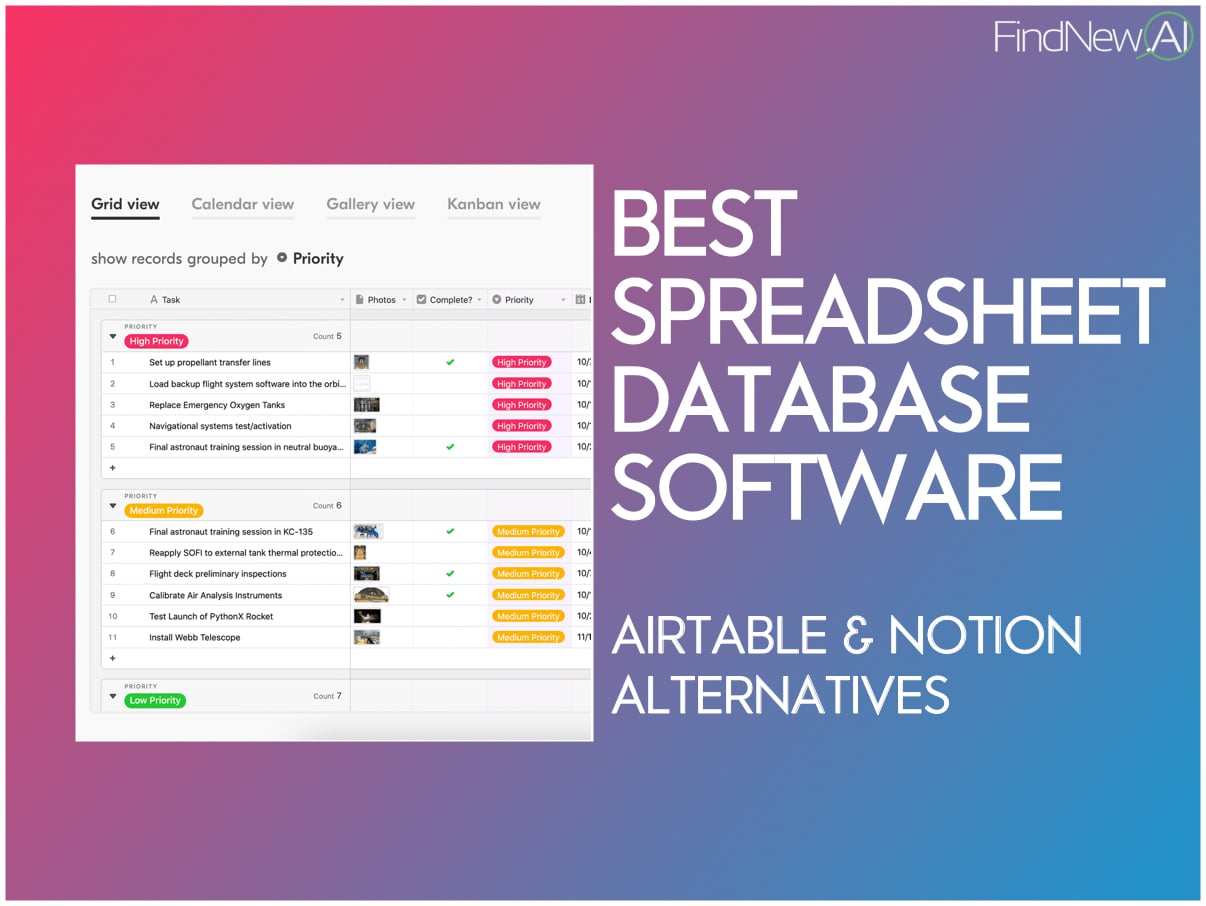
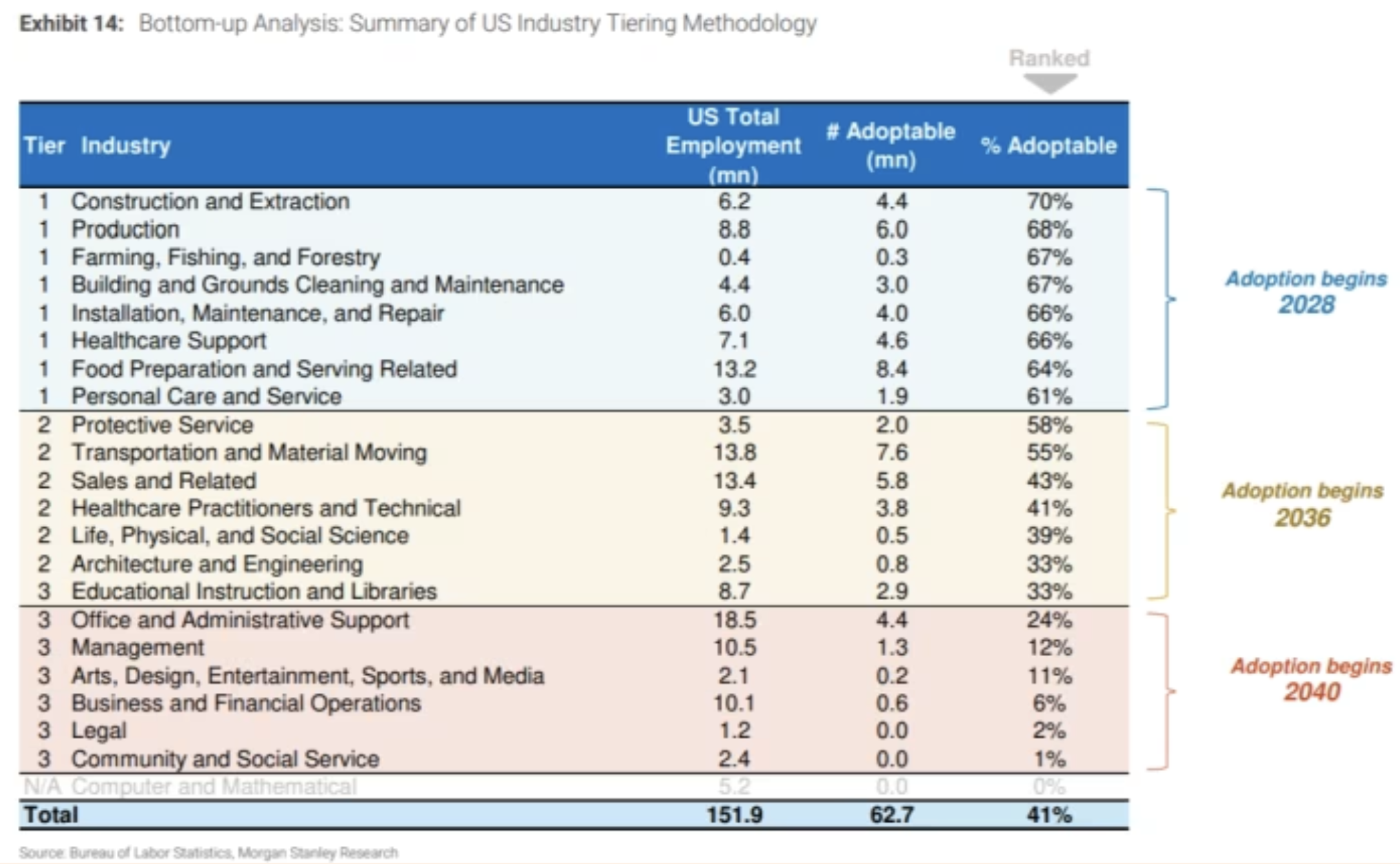
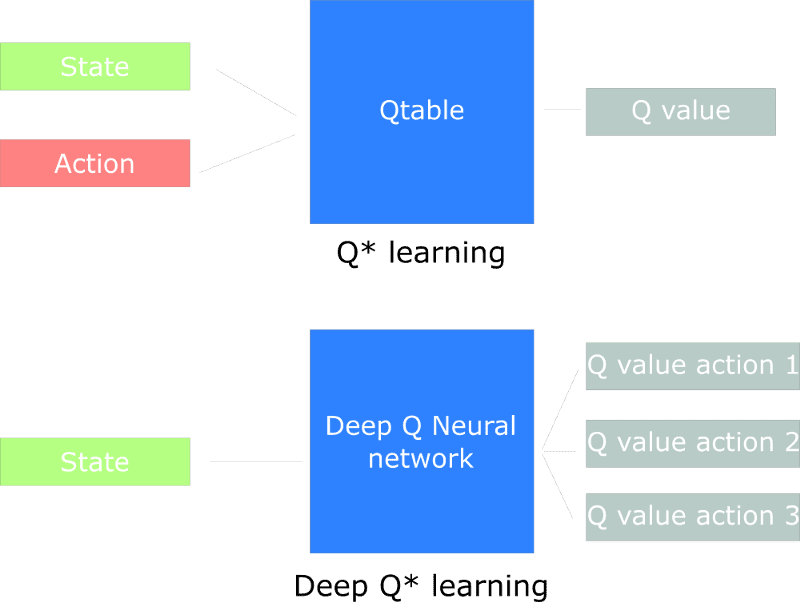
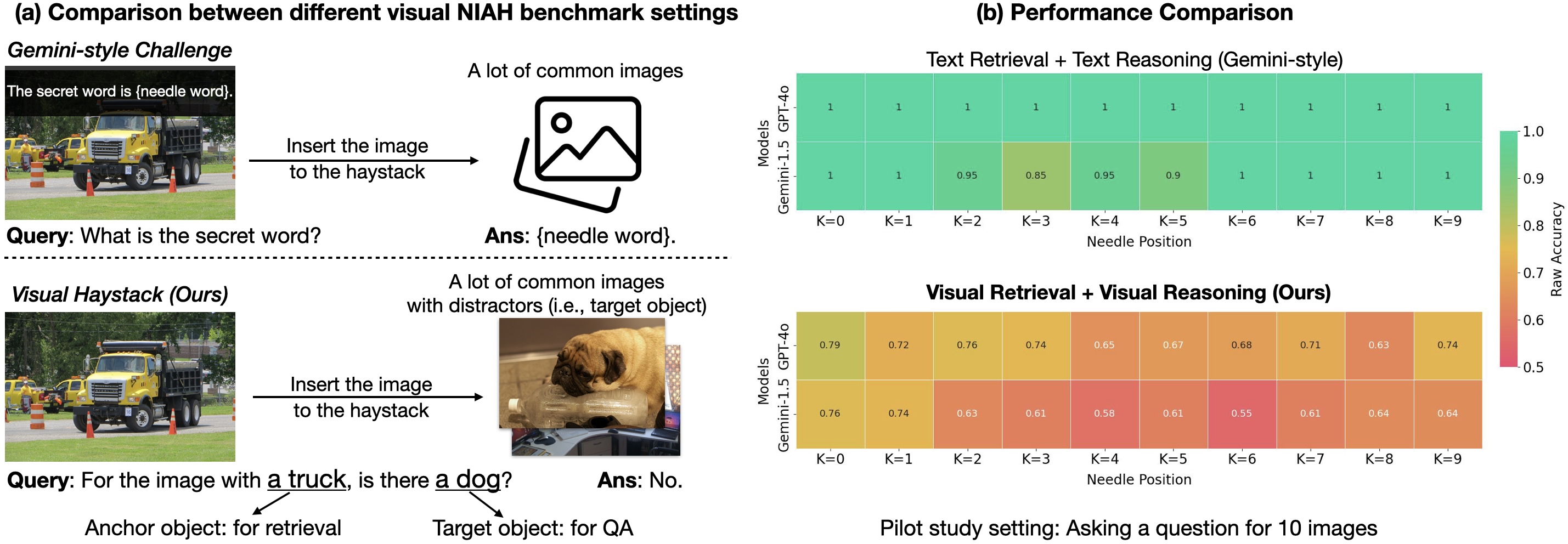
Cooperatives on Socio-data Pools as per "Closures"
Examples on data-revolving cooperative communities include cooperatives of music artists, video producers, and gig workers. The income is not a subsidy, but rather the result of individual economic activity channeled through exchanges that aggregate the data of producers and workers, thereby turning individuals into data entrepreneurs. Total assets reported by this sector have increased somewhat despite the decrease in numbers, and liabilities have generally declined substantially . The average debt-to-asset ratio remains low and unchanged since 1989, suggesting good financial health for data co-operatives . The large increase in revenues appears to be offset by higher expenses, with the 1996 surplus much lower ( 21 percent) than that reported in 1989. Employment increased between 1989 and 1996, with a substantially larger wage bill re-ported, while capital investment rose from $5.045 million in 1989 to $8 million in 1996. The 1996 financial statistics for each type of recreational co-operatives are signs of such small businesses being on the verge of becoming mid-sized . Mathematical functions' analyses demonstrate that set of closures on data pool integrations have already passed the threshold of merely remaining coordinate-less asymptotes . Consequently it has of course never been possible to give a full and final account of geometry as such working on the data pooled thereof .One of the groups I am aiming at, is future teachers of mathematics. All too often the geometry which goes into the syllabus for teacher-students present the material as pedantic and formalistic, suppressing the very powerful and dynamic character of this old - and yet so young! - field. A field of mathematical insight, research, history and source of artistic inspiration. And not least important, a foundation for our common cultural heritage. Reviewing the programme specifications, it was possible to broadly gauge the scope, breadth and depth of subject content taught within each qualification4, which could then be further examined within the subsequent examination paper analysis; including whether papers assess one particular area of mathematics or assess a range of mathematical topics. Any comparisons of assessment item demand need to be contextualised by the content breadth and depth of the respective programme. For example, it might be expected that an international programme focusing in-depth on a particular topic of mathematics may (though not necessarily) pose more questions, and of a more complex nature, on a given topic than those programmes designed to test a broad range of mathematics topics in such Co-ops .
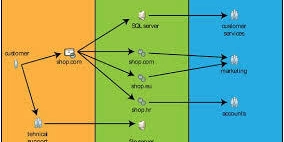
Examples on data-revolving cooperative communities include cooperatives of music artists, video producers, and gig workers. The income is not a subsidy, but rather the result of individual economic activity channeled through exchanges that aggregate the data of producers and workers, thereby turning individuals into data entrepreneurs. Total assets reported by this sector have increased somewhat despite the decrease in numbers, and liabilities have generally declined substantially . The average debt-to-asset ratio remains low and unchanged since 1989, suggesting good financial health for data co-operatives . The large increase in revenues appears to be offset by higher expenses, with the 1996 surplus much lower ( 21 percent) than that reported in 1989. Employment increased between 1989 and 1996, with a substantially larger wage bill re-ported, while capital investment rose from $5.045 million in 1989 to $8 million in 1996.
The 1996 financial statistics for each type of recreational co-operatives are signs of such small businesses being on the verge of becoming mid-sized . Mathematical functions' analyses demonstrate that set of closures on data pool integrations have already passed the threshold of merely remaining coordinate-less asymptotes . Consequently it has of course never been possible to give a full and final account of geometry as such working on the data pooled thereof .One of the groups I am aiming at, is future teachers of mathematics. All too often the geometry which goes into the syllabus for teacher-students present the material as pedantic and formalistic, suppressing the very powerful and dynamic character of this old - and yet so young! - field. A field of mathematical insight, research, history and source of artistic inspiration. And not least important, a foundation for our common cultural heritage. Reviewing the programme specifications, it was possible to broadly gauge the scope, breadth and depth of subject content taught within each qualification4, which could then be further examined within the subsequent examination paper analysis; including whether papers assess one particular area of mathematics or assess a range of mathematical topics. Any comparisons of assessment item demand need to be contextualised by the content breadth and depth of the respective programme. For example, it might be expected that an international programme focusing in-depth on a particular topic of mathematics may (though not necessarily) pose more questions, and of a more complex nature, on a given topic than those programmes designed to test a broad range of mathematics topics in such Co-ops .
























































































































































![[The AI Show Episode 145]: OpenAI Releases o3 and o4-mini, AI Is Causing “Quiet Layoffs,” Executive Order on Youth AI Education & GPT-4o’s Controversial Update](https://www.marketingaiinstitute.com/hubfs/ep%20145%20cover.png)
















































































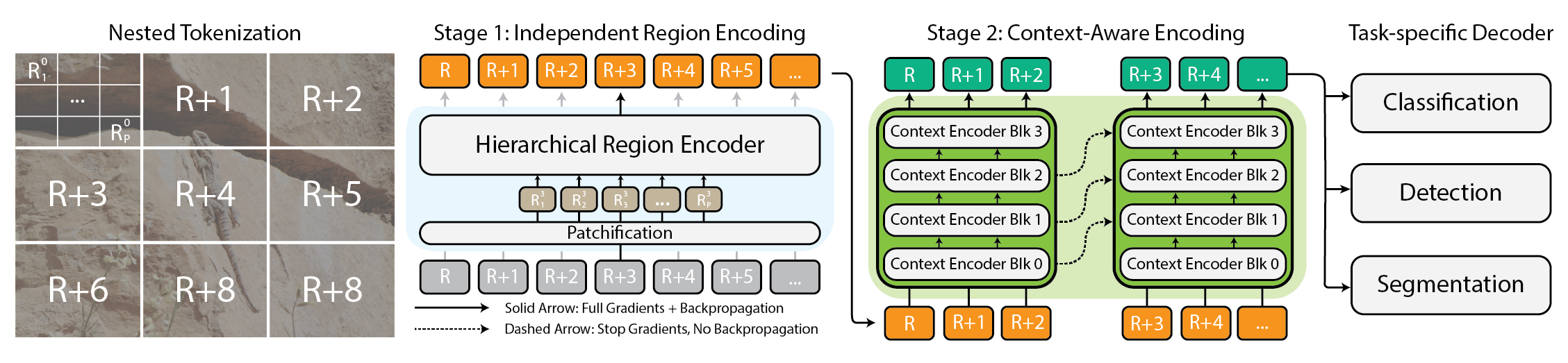
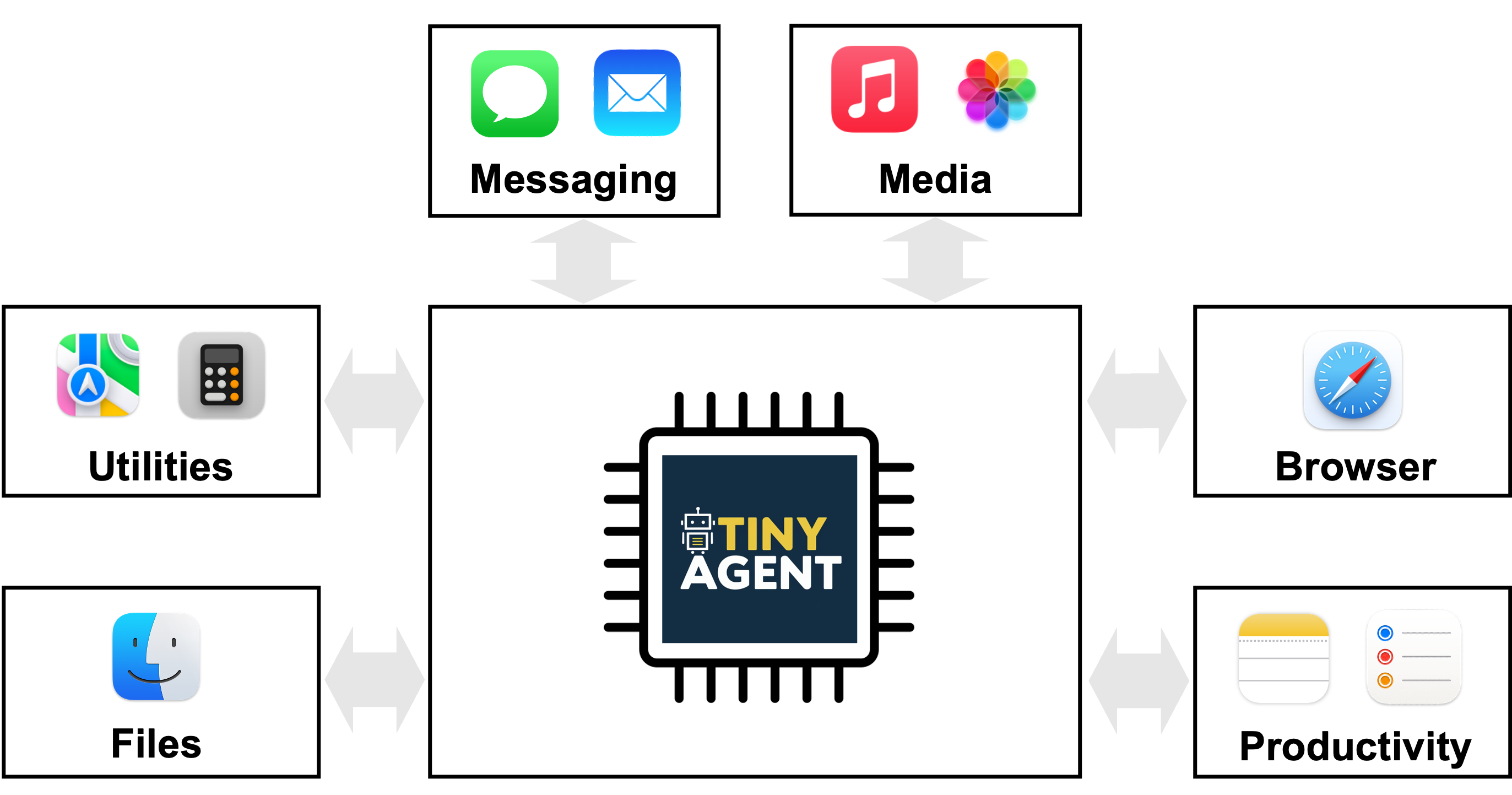















































































































































_NicoElNino_Alamy.jpg?width=1280&auto=webp&quality=80&disable=upscale#)























































































![Craft adds Readwise integration for working with book notes and highlights [50% off]](https://i0.wp.com/9to5mac.com/wp-content/uploads/sites/6/2025/04/craft3.jpg.png?resize=1200%2C628&quality=82&strip=all&ssl=1)















![Apple Restructures Global Affairs and Apple Music Teams [Report]](https://www.iclarified.com/images/news/97162/97162/97162-640.jpg)
![New iPhone Factory Goes Live in India, Another Just Days Away [Report]](https://www.iclarified.com/images/news/97165/97165/97165-640.jpg)