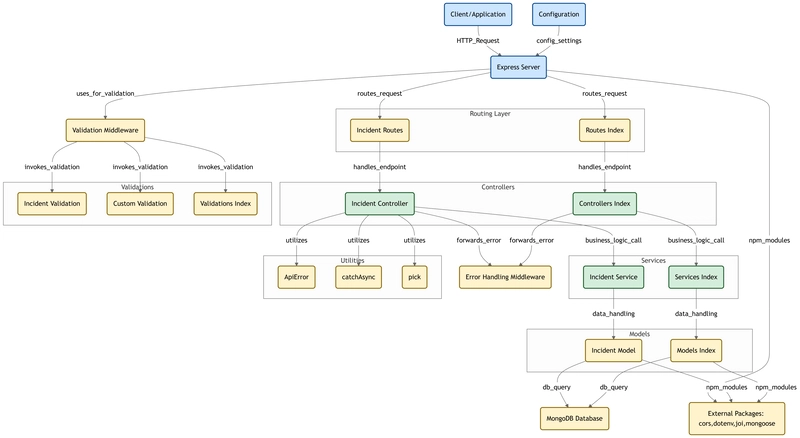
More Math Algorithms in Python Without Libraries
In today's post, we're diving into two classic number theory problems that strengthen your grasp of math logic using plain Python—no math, numpy, or sympy. Problem 1: Get All Unique Prime Factors of an Integer Create a Python function called get_prime_factors(n) that returns all unique prime factors of an integer n in a sorted list. Do not use built-in libraries. Assume time complexity around O(n). Breakdown: If n

In today's post, we're diving into two classic number theory problems that strengthen your grasp of math logic using plain Python—no math, numpy, or sympy.
Problem 1: Get All Unique Prime Factors of an Integer
Create a Python function called
get_prime_factors(n)that returns all unique prime factors of an integernin a sorted list. Do not use built-in libraries. Assume time complexity around O(n).
Breakdown:
- If
n <= 1, return an empty list (no factors). - First, handle the even number case (
2) explicitly. - Then, check odd numbers up to the square root of
n. - Any number remaining after that loop is a prime factor.
- Use a custom sort (without
sorted()orsort()) to return factors in ascending order.
Solution:
def get_prime_factors(n):
if n <= 1:
return []
prime_factors = []
if n % 2 == 0:
prime_factors.append(2)
while n % 2 == 0:
n //= 2
for i in range(3, int(n**0.5) + 1, 2):
if n % i == 0:
prime_factors.append(i)
while n % i == 0:
n //= i
if n > 2:
prime_factors.append(n)
# Manual sorting (selection sort style)
sorted_prime_factors = []
while prime_factors:
min_value = prime_factors[0]
for value in prime_factors:
if value < min_value:
min_value = value
sorted_prime_factors.append(min_value)
prime_factors = [value for value in prime_factors if value != min_value]
return sorted_prime_factors
pass
Problem 2: Check If Two Numbers Are Co-Prime
You are given two integers
aandb. Write a functionare_coprime(a, b)that returnsTrueif they are co-prime, i.e., their greatest common divisor (GCD) is 1. Do not use built-in libraries.
Breakdown:
- Use the classic Euclidean algorithm to compute the GCD manually.
- Two numbers are co-prime if
gcd(a, b) == 1. - Handle edge cases like negative or zero inputs.
Solution:
def gcd(a, b):
while b != 0:
a, b = b, a % b
return a
def are_coprime(a, b):
if a <= 0 or b <= 0:
return False
return gcd(a, b) == 1
pass
These examples show how to build up number-theoretic algorithms from scratch. Practicing such problems sharpens your algorithmic reasoning and boosts your confidence for interviews and real-world coding alike!




















































_courtesy_VERTICAL.jpg)











































































































![[The AI Show Episode 145]: OpenAI Releases o3 and o4-mini, AI Is Causing “Quiet Layoffs,” Executive Order on Youth AI Education & GPT-4o’s Controversial Update](https://www.marketingaiinstitute.com/hubfs/ep%20145%20cover.png)























































































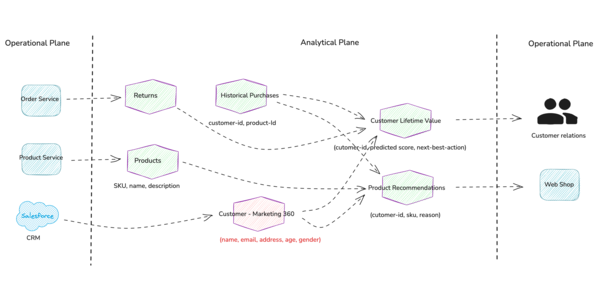





![[DEALS] Mail Backup X Individual Edition: Lifetime Subscription (72% off) & Other Deals Up To 98% Off – Offers End Soon!](https://www.javacodegeeks.com/wp-content/uploads/2012/12/jcg-logo.jpg)






















































































































![Google reveals NotebookLM app for Android & iPhone, coming at I/O 2025 [Gallery]](https://i0.wp.com/9to5google.com/wp-content/uploads/sites/4/2025/05/NotebookLM-Android-iPhone-6-cover.jpg?resize=1200%2C628&quality=82&strip=all&ssl=1)




















































































































![Apple Reports Q2 FY25 Earnings: $95.4 Billion in Revenue, $24.8 Billion in Net Income [Chart]](https://www.iclarified.com/images/news/97188/97188/97188-640.jpg)